Portal:Acoustics
Jump to navigation
Jump to search
Introduction
Artificial omni-directional sound source in an anechoic chamber
Acoustics is the branch of physics that deals with the study of all mechanical waves in gases, liquids, and solids including topics such as vibration, sound, ultrasound and infrasound. A scientist who works in the field of acoustics is an acoustician while someone working in the field of acoustics technology may be called an acoustical engineer. The application of acoustics is present in almost all aspects of modern society with the most obvious being the audio and noise control industries.
Hearing is one of the most crucial means of survival in the animal world, and speech is one of the most distinctive characteristics of human development and culture. Accordingly, the science of acoustics spreads across many facets of human society—music, medicine, architecture, industrial production, warfare and more. Likewise, animal species such as songbirds and frogs use sound and hearing as a key element of mating rituals or marking territories. Art, craft, science and technology have provoked one another to advance the whole, as in many other fields of knowledge. Robert Bruce Lindsay's 'Wheel of Acoustics' is a well accepted overview of the various fields in acoustics.
Selected general articles
String resonance occurs on string instruments. Strings or parts of strings may resonate at their fundamental or overtone frequencies when other strings are sounded. For example, an A string at 440 Hz will cause an E string at 330 Hz to resonate, because they share an overtone of 1320 Hz (3rd overtone of A and 4th overtone of E).
Electric guitars can have string trees near the tuning pegs to mute this type of reverberation. The string length behind the bridge also must be as short as possible to prevent the resonance.
String resonance is a factor in the timbre of a string instrument. Tailed bridge guitars like the Fender Jaguar differ in timbre from guitars with short bridges, because of their (extended) floating bridge. The Japanese Koto is also an example of an instrument with occurring string resonance. Read more...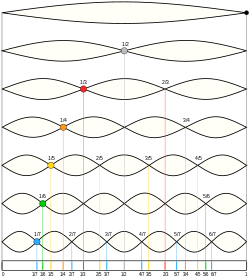
The nodes of a vibrating string are harmonics.
A harmonic is any member of the harmonic series. The term is employed in various disciplines, including music, physics, acoustics, electronic power transmission, radio technology, and other fields. It is typically applied to repeating signals, such as sinusoidal waves. A harmonic of such a wave is a wave with a frequency that is a positive integer multiple of the frequency of the original wave, known as the fundamental frequency. The original wave is also called the 1st harmonic, the following harmonics are known as higher harmonics. As all harmonics are periodic at the fundamental frequency, the sum of harmonics is also periodic at that frequency. For example, if the fundamental frequency is 50 Hz, a common AC power supply frequency, the frequencies of the first three higher harmonics are 100 Hz (2nd harmonic), 150 Hz (3rd harmonic), 200 Hz (4th harmonic) and any addition of waves with these frequencies is periodic at 50 Hz.
In music, harmonics are used on string instruments and wind instruments as a way of producing sound on the instrument, particularly to play higher notes and, with strings, obtain notes that have a unique sound quality or "tone colour". On strings, harmonics that are bowed have a "glassy", pure tone. On stringed instruments, harmonics are played by touching (but not fully pressing down the string) at an exact point on the string while sounding the string (plucking, bowing, etc.); this allows the harmonic to sound, a pitch which is always higher than the fundamental frequency of the string. Read more...
Hermann Ludwig Ferdinand von Helmholtz (August 31, 1821 – September 8, 1894) was a German physician and physicist who made significant contributions in several scientific fields. The largest German association of research institutions, the Helmholtz Association, is named after him.
In physiology and psychology, he is known for his mathematics of the eye, theories of vision, ideas on the visual perception of space, color vision research, and on the sensation of tone, perception of sound, and empiricism in the physiology of perception.
In physics, he is known for his theories on the conservation of energy, work in electrodynamics, chemical thermodynamics, and on a mechanical foundation of thermodynamics. Read more...
In physics, a standing wave – also known as a stationary wave – is a wave which oscillates in time but whose peak amplitude profile does not move in space. The peak amplitude of the wave oscillations at any point in space is constant with time, and the oscillations at different points throughout the wave are in phase. The locations at which the amplitude is minimum are called nodes, and the locations where the amplitude is maximum are called antinodes.
Standing waves were first noticed by Michael Faraday in 1831. Faraday observed standing waves on the surface of a liquid in a vibrating container. Franz Melde coined the term "standing wave" (German: stehende Welle or Stehwelle) around 1860 and demonstrated the phenomenon in his classic experiment with vibrating strings.
This phenomenon can occur because the medium is moving in the opposite direction to the wave, or it can arise in a stationary medium as a result of interference between two waves traveling in opposite directions. The most common cause of standing waves is the phenomenon of resonance, in which standing waves occur inside a resonator due to interference between waves reflected back and forth at the resonator's resonant frequency. Read more...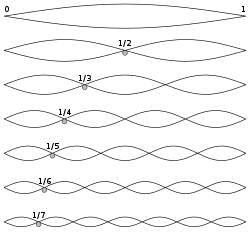
Vibrational modes of an ideal string, dividing the string length into integer divisions, producing harmonic partials f, 2f, 3f, 4f, etc. (where f means fundamental frequency).
An overtone is any frequency greater than the fundamental frequency of a sound. Using the model of Fourier analysis, the fundamental and the overtones together are called partials. Harmonics, or more precisely, harmonic partials, are partials whose frequencies are numerical integer multiples of the fundamental (including the fundamental, which is 1 times itself). These overlapping terms are variously used when discussing the acoustic behavior of musical instruments. (See etymology below.) The model of Fourier analysis provides for the inclusion of inharmonic partials, which are partials whose frequencies are not whole-number ratios of the fundamental (such as 1.1 or 2.14179).
When a resonant system such as a blown pipe or plucked string is excited, a number of overtones may be produced along with the fundamental tone. In simple cases, such as for most musical instruments, the frequencies of these tones are the same as (or close to) the harmonics. Examples of exceptions include the circular drum, – a timpani whose first overtone is about 1.6 times its fundamental resonance frequency, gongs and cymbals, and brass instruments. The human vocal tract is able to produce highly variable amplitudes of the overtones, called formants, which define different vowels. Read more...
Franz Emil Melde (March 11, 1832 in Großenlüder near Fulda – March 17, 1901 in Marburg) was a German physicist and professor. A graduate of the University of Marburg under Christian Ludwig Gerling, he later taught there, focusing primarily on acoustics, also making contributions to fields including fluid mechanics and meteorology. He began in 1860 as Gerling's assistant at the University's Mathematical and Physical Institute, succeeding him in 1864.
Standing waves were first discovered by Melde, who coined the term "standing wave" (stehende Welle) around 1860. What is known as "Melde's experiment", "a lecture-room standby", demonstrates standing waves and their patterns on a string, is used to measure the speed of transverse wave, and to determine the effect of tension, length, and mass on the transverse waves of a string. In 1859 Melde generated parametric oscillations in a string by employing a tuning fork to periodically vary the tension at twice the resonance frequency of the monochord string.
He was a member of the Landsmannschaft fraternity. In 1885 he was elected to the Academy of Sciences Leopoldina and in 1893 received a silver medal at the Chicago Columbian Exposition. Read more...
The Bark scale is a psychoacoustical scale proposed by Eberhard Zwicker in 1961. It is named after Heinrich Barkhausen who proposed the first subjective measurements of loudness. One definition of the term is "...a frequency scale on which equal distances correspond with perceptually equal distances. Above about 500 Hz this scale is more or less equal to a logarithmic frequency axis. Below 500 Hz the Bark scale becomes more and more linear."
The scale ranges from 1 to 24 and corresponds to the first 24 critical bands of hearing.
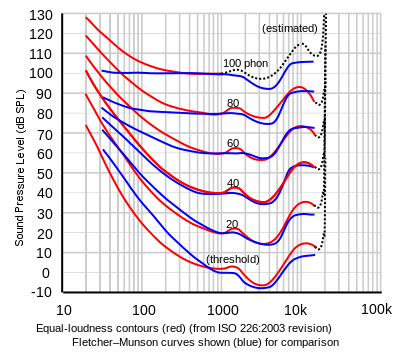
It is related to, but somewhat less popular than, the mel scale, a perceptual scale of pitches judged by listeners to be equal in distance from one another. Read more...
ISO equal-loudness contours with frequency in Hz.
An equal-loudness contour is a measure of sound pressure (dB SPL), over the frequency spectrum, for which a listener perceives a constant loudness when presented with pure steady tones. The unit of measurement for loudness levels is the phon, and is arrived at by reference to equal-loudness contours. By definition, two sine waves of differing frequencies are said to have equal-loudness level measured in phons if they are perceived as equally loud by the average young person without significant hearing impairment.
Equal-loudness contours are often referred to as "Fletcher-Munson" curves, after the earliest researchers, but those studies have been superseded and incorporated into newer standards. The definitive curves are those defined in the international standard ISO 226:2003, which are based on a review of modern determinations made in various countries. Read more...
Symphony Hall, Birmingham, an example of the application of architectural acoustics.
Architectural acoustics (also known as room acoustics and building acoustics) is the science and engineering of achieving a good sound within a building and is a branch of acoustical engineering. The first application of modern scientific methods to architectural acoustics was carried out by Wallace Sabine in the Fogg Museum lecture room who then applied his new found knowledge to the design of Symphony Hall, Boston.
Architectural acoustics can be about achieving good speech intelligibility in a theatre, restaurant or railway station, enhancing the quality of music in a concert hall or recording studio, or suppressing noise to make offices and homes more productive and pleasant places to work and live in. Architectural acoustic design is usually done by acoustic consultants. Read more...
A harmonic series is the sequence of sounds—pure tones, represented by sinusoidal waves—in which the frequency of each sound is an integer multiple of the fundamental, the lowest frequency.
Pitched musical instruments are often based on an acoustic resonator such as a string or a column of air, which oscillates at numerous modes simultaneously. At the frequencies of each vibrating mode, waves travel in both directions along the string or air column, reinforcing and canceling each other to form standing waves. Interaction with the surrounding air causes audible sound waves, which travel away from the instrument. Because of the typical spacing of the resonances, these frequencies are mostly limited to integer multiples, or harmonics, of the lowest frequency, and such multiples form the harmonic series (see harmonic series (mathematics)).
The musical pitch of a note is usually perceived as the lowest partial present (the fundamental frequency), which may be the one created by vibration over the full length of the string or air column, or a higher harmonic chosen by the player. The musical timbre of a steady tone from such an instrument is strongly affected by the relative strength of each harmonic. Read more...
The mel scale, named by Stevens, Volkmann, and Newman in 1937,
is a perceptual scale of pitches judged by listeners to be equal in distance from one another. The reference point between this scale and normal frequency measurement is defined by assigning a perceptual pitch of 1000 mels to a 1000 Hz tone, 40 dB above the listener's threshold. Above about 500 Hz, increasingly large intervals are judged by listeners to produce equal pitch increments. As a result, four octaves on the hertz scale above 500 Hz are judged to comprise about two octaves on the mel scale. The name mel comes from the word melody to indicate that the scale is based on pitch comparisons. Read more...- Werner Meyer-Eppler (30 April 1913 – 8 July 1960), was a Belgian-born German physicist, experimental acoustician, phoneticist and information theorist.
Meyer-Eppler was born in Antwerp. He studied mathematics, physics, and chemistry, first at the University of Cologne and then in Bonn, from 1936 until 1939, when he received a doctorate in Physics. From 1942 to 1945 he was a scientific assistant at the Physics Institute of the University of Bonn. From the time of his habilitation on 16 September 1942, he was also Lecturer in Experimental Physics. After the end of the war, Meyer-Eppler turned his attention increasingly to phonetics and speech synthesis. In 1947 he was recruited by Paul Menzerath to the faculty of the Phonetic Institute of the University of Bonn, where he became Scientific Assistant on 1 April 1949. During this time, Meyer-Eppler published essays on synthetic language production and presented American inventions like the Coder, the Vocoder, the Visible Speech Machine. He contributed to the development of the electrolarynx, which is still used today for the speech-impaired (Ungeheuer 1992; Diesterhöft 2003).
In 1949, Meyer-Eppler published a book promoting the idea of producing music by purely electronic means (Meyer-Eppler 1949), and in 1951 joined the sound engineer/composer Robert Beyer and the composer/musicologist/journalist Herbert Eimert in a successful proposal to the Nordwestdeutscher Rundfunk (NWDR) for the establishment of an electronic-music studio in Cologne. After two years of work, it was officially opened with a broadcast lecture-concert on 26 May 1953, and was to become the most important such studio in Europe. Read more...
Marin Mersenne, Marin Mersennus or le Père Mersenne (French: [mɛʀsɛn]; 8 September 1588 – 1 September 1648) was a French polymath, whose works touched a wide variety of fields. He is perhaps best known today among mathematicians for Mersenne prime numbers, those which can be written in the form {{{1}}} for some integer n. He also developed Mersenne's laws, which describe the harmonics of a vibrating string (such as may be found on guitars and pianos), and his seminal work on music theory, Harmonie universelle, for which he is referred to as the "father of acoustics". Mersenne, an ordained priest, had many contacts in the scientific world and has been called "the center of the world of science and mathematics during the first half of the 1600s" and, because of his ability to make connections between people and ideas, "the post-box of Europe". He was also a member of the Minim religious order and wrote and lectured on theology and philosophy. Read more...
Infrasound arrays at infrasound monitoring station in Qaanaaq, Greenland
Infrasound, sometimes referred to as low-frequency sound, is sound that is lower in frequency than 20 Hz or cycles per second, the "normal" limit of human hearing. Hearing becomes gradually less sensitive as frequency decreases, so for humans to perceive infrasound, the sound pressure must be sufficiently high. The ear is the primary organ for sensing infrasound, but at higher intensities it is possible to feel infrasound vibrations in various parts of the body.
The study of such sound waves is sometimes referred to as infrasonics, covering sounds beneath 20 Hz down to 0.1 Hz and rarely to 0.001 Hz. People use this frequency range for monitoring earthquakes, charting rock and petroleum formations below the earth, and also in ballistocardiography and seismocardiography to study the mechanics of the heart.
Infrasound is characterized by an ability to get around obstacles with little dissipation. In music, acoustic waveguide methods, such as a large pipe organ or, for reproduction, exotic loudspeaker designs such as transmission line, rotary woofer, or traditional subwoofer designs can produce low-frequency sounds, including near-infrasound. Subwoofers designed to produce infrasound are capable of sound reproduction an octave or more below that of most commercially available subwoofers, and are often about 10 times the size. Read more...
In speech science and phonetics, a formant is the spectral shaping that results from an acoustic resonance of the human vocal tract. However, in acoustics, the definition of a formant differs slightly as it is defined as a peak, or local maximum, in the spectrum. For harmonic sounds, with this definition, it is therefore the harmonic partial that is augmented by a resonance. The difference between these two definitions resides in whether "formants" characterise the production mechanisms of a sound or the produced sound itself. In practice, the frequency of a spectral peak can differ from the associated resonance frequency when, for instance, harmonics are not aligned with the resonance frequency. In most cases, this subtle difference is irrelevant and, in phonetics, formant can mean either a resonance or the spectral maximum that the resonance produces. Formants are often measured as amplitude peaks in the frequency spectrum of the sound, using a spectrogram (in the figure) or a spectrum analyzer and, in the case of the voice, this gives an estimate of the vocal tract resonances. In vowels spoken with a high fundamental frequency, as in a female or child voice, however, the frequency of the resonance may lie between the widely spaced harmonics and hence no corresponding peak is visible.
A room can be said to have formants characteristic of that particular room, due to the way sound reflects from its walls and objects. Room formants of this nature reinforce themselves by emphasizing specific frequencies and absorbing others, as exploited, for example, by Alvin Lucier in his piece I Am Sitting in a Room. Read more...
A drum produces sound via a vibrating membrane.
In physics, sound is a vibration that typically propagates as an audible wave of pressure, through a transmission medium such as a gas, liquid or solid.
In human physiology and psychology, sound is the reception of such waves and their perception by the brain. Humans can only hear sound waves as distinct pitches when the frequency lies between about 20 Hz and 20 kHz. Sound waves above 20 kHz are known as ultrasound and is not perceptible by humans. Sound waves below 20 Hz are known as infrasound. Different animal species have varying hearing ranges. Read more...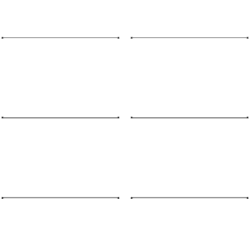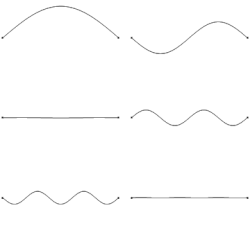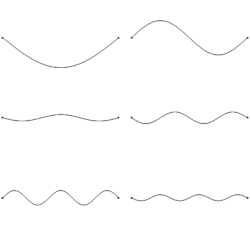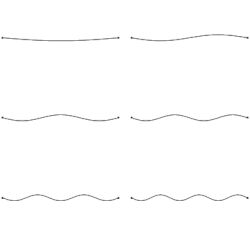
Vibration, standing waves in a string. The fundamental and the first 5 overtones in the harmonic series.
A vibration in a string is a wave. Resonance causes a vibrating string to produce a sound with constant frequency, i.e. constant pitch. If the length or tension of the string is correctly adjusted, the sound produced is a musical tone. Vibrating strings are the basis of string instruments such as guitars, cellos, and pianos. Read more...
The bottom waveform is missing the fundamental frequency, 100 hertz, and the second harmonic, 200 hertz. The periodicity is nevertheless clear when compared to the full-spectrum waveform on top.
A harmonic sound is said to have a missing fundamental, suppressed fundamental, or phantom fundamental when its overtones suggest a fundamental frequency but the sound lacks a component at the fundamental frequency itself.
The brain perceives the pitch of a tone not only by its fundamental frequency, but also by the periodicity implied by the relationship between the higher harmonics; we may perceive the same pitch (perhaps with a different timbre) even if the fundamental frequency is missing from a tone.
For example, when a note (that is not a pure tone) has a pitch of 100 Hz, it will consist of frequency components that are integer multiples of that value (e.g. 100, 200, 300, 400, 500.... Hz). However, smaller loudspeakers may not produce low frequencies, and so in our example, the 100 Hz component may be missing. Nevertheless, a pitch corresponding to the fundamental may still be heard. Read more...
In music, inharmonicity is the degree to which the frequencies of overtones (also known as partials or partial tones) depart from whole multiples of the fundamental frequency (harmonic series).
Acoustically, a note perceived to have a single distinct pitch in fact contains a variety of additional overtones. Many percussion instruments, such as cymbals, tam-tams, and chimes, create complex and inharmonic sounds.
However, in stringed instruments such as the piano, violin, and guitar, or in some Indian drums such as tabla, the overtones are close to—or in some cases, quite exactly—whole number multiples of the fundamental frequency. Any departure from this ideal harmonic series is known as inharmonicity. The less elastic the strings are (that is, the shorter, thicker, smaller tension or stiffer they are), the more inharmonicity they exhibit. Read more...
In acoustics, a beat is an interference pattern between two sounds of slightly different frequencies, perceived as a periodic variation in volume whose rate is the difference of the two frequencies.
When tuning instruments that can produce sustained tones, beats can be readily recognized. Tuning two tones to a unison will present a peculiar effect: when the two tones are close in pitch but not identical, the difference in frequency generates the beating. The volume varies like in a tremolo as the sounds alternately interfere constructively and destructively. As the two tones gradually approach unison, the beating slows down and may become so slow as to be imperceptible. As the two tones get further apart, their beat frequency starts to approach the range of human pitch perception, the beating starts to sound like a note, and a combination tone is produced. This combination tone can also be referred to as a missing fundamental, as the beat frequency of any two tones is equivalent to the frequency of their implied fundamental frequency. Read more...
Thomas Young FRS (13 June 1773 – 10 May 1829) was a British polymath and physician. Young made notable scientific contributions to the fields of vision, light, solid mechanics, energy, physiology, language, musical harmony, and Egyptology. He "made a number of original and insightful innovations" in the decipherment of Egyptian hieroglyphs (specifically the Rosetta Stone) before Jean-François Champollion eventually expanded on his work. He was mentioned by, among others, William Herschel, Hermann von Helmholtz, James Clerk Maxwell, and Albert Einstein. Young has been described as "The Last Man Who Knew Everything". Read more...
The Fletcher–Munson curves are one of many sets of equal-loudness contours for the human ear, determined experimentally by Harvey Fletcher and Wilden A. Munson, and reported in a 1933 paper entitled "Loudness, its definition, measurement and calculation" in the Journal of the Acoustical Society of America. Read more...
John William Strutt, 3rd Baron Rayleigh, OM, PC, PRS (/ˈreɪli/; 12 November 1842 – 30 June 1919), was a British scientist who made extensive contributions to both theoretical and experimental physics. He spent all of his academic career at the University of Cambridge. Among many honours, he received the 1904 Nobel Prize in Physics "for his investigations of the densities of the most important gases and for his discovery of argon in connection with these studies." He served as President of the Royal Society from 1905 to 1908 and as Chancellor of the University of Cambridge from 1908 to 1919.
Rayleigh provided the first theoretical treatment of the elastic scattering of light by particles much smaller than the light's wavelength, a phenomenon now known as "Rayleigh scattering", which notably explains why the sky is blue. He studied and described transverse surface waves in solids, now known as "Rayleigh waves". He contributed extensively to fluid dynamics, with concepts such as the Rayleigh number (a dimensionless number associated with natural convection), Rayleigh flow, the Rayleigh–Taylor instability, and Rayleigh's criterion for the stability of Taylor–Couette flow. He also formulated the circulation theory of aerodynamic lift. In optics, Rayleigh proposed a well known criterion for angular resolution. His derivation of the Rayleigh–Jeans law for classical black-body radiation later played an important role in birth of quantum mechanics (see Ultraviolet catastrophe). Rayleigh's textbook The Theory of Sound (1877) is still used today by acousticians and engineers. Read more...
In music, the undertone series or subharmonic series is a sequence of notes that results from inverting the intervals of the overtone series. While overtones naturally occur with the physical production of music on instruments, undertones must be produced in unusual ways. While the overtone series is based upon arithmetic multiplication of frequencies, resulting in a harmonic series, the undertone series is based on arithmetic division.
The hybrid term subharmonic is used in music in a few different ways. In its pure sense, the term subharmonic refers strictly to any member of the subharmonic series (1/1, 1/2, 1/3, 1/4, etc.). When the subharmonic series is used to refer to frequency relationships, it is written with f representing some highest known reference frequency (f/1, f/2, f/3, f/4, etc.). The complex tones of acoustic instruments do not produce partials that resemble the subharmonic series. However, such tones can be produced artificially with audio software and electronics. Subharmonics can be contrasted with harmonics. While harmonics can "...occur in any nonlinear system", there are "...only fairly restricted conditions" that will lead to the "nonlinear phenomenon known as subharmonic generation".
One way to define subharmonics is that they are "...integral submultiples of the fundamental (driving) frequency".
In a second sense, subharmonic does not relate to the subharmonic series, but instead describes an instrumental technique for lowering the pitch of an acoustic instrument below what would be expected for the resonant frequency of that instrument, such as a violin string that is driven and damped by increased bow pressure to produce a fundamental frequency lower than the normal pitch of the same open string. The human voice can also be forced into a similar driven resonance, also called “undertone singing” (which similarly has nothing to do with the undertone series), to extend the range of the voice below what is normally available. However, the frequency relationships of the component partials of the tone produced by the acoustic instrument or voice played in such a way still resemble the harmonic series, not the subharmonic series. In this sense, subharmonic is a term created by reflection from the second sense of the term harmonic, which in that sense refers to an instrumental technique for making an instrument's pitch seem higher than normal by eliminating some lower partials by damping the resonator at the antinodes of vibration of those partials (such as placing a finger lightly on a string at certain locations). Read more...
An anechoic chamber, showing acoustic damping tiles used for sound absorption.
Soundproofing is any means of reducing the sound pressure with respect to a specified sound source and receptor. There are several basic approaches to reducing sound: increasing the distance between source and receiver, using noise barriers to reflect or absorb the energy of the sound waves, using damping structures such as sound baffles, or using active antinoise sound generators.
Two distinct soundproofing problems may need to be considered when designing acoustic treatments - to improve the sound within a room (see reverberation), and reduce sound leakage to/from adjacent rooms or outdoors (see sound transmission class and sound reduction index). Acoustic quieting and noise control can be used to limit unwanted noise. Soundproofing can suppress unwanted indirect sound waves such as reflections that cause echoes and resonances that cause reverberation. Soundproofing can reduce the transmission of unwanted direct sound waves from the source to an involuntary listener through the use of distance and intervening objects in the sound path. Read more...- Reverberation, in psychoacoustics and acoustics, is a persistence of sound after the sound is produced. A reverberation, or reverb, is created when a sound or signal is reflected causing a large number of reflections to build up and then decay as the sound is absorbed by the surfaces of objects in the space – which could include furniture, people, and air. This is most noticeable when the sound source stops but the reflections continue, decreasing in amplitude, until they reach zero amplitude.
Reverberation is frequency dependent: the length of the decay, or reverberation time, receives special consideration in the architectural design of spaces which need to have specific reverberation times to achieve optimum performance for their intended activity. In comparison to a distinct echo, that is detectable at a minimum of 50 to 100 ms after the previous sound, reverberation is the occurrence of reflections that arrive in a sequence of less than approximately 50 ms. As time passes, the amplitude of the reflections gradually reduces to non-noticeable levels. Reverberation is not limited to indoor spaces as it exists in forests and other outdoor environments where reflection exists.
Reverberation occurs naturally when a person sings, talks, or plays an instrument acoustically in a hall or performance space with sound-reflective surfaces. The sound of reverberation is often electronically added to the vocals of singers and to musical instruments. This is done in both live sound systems and sound recordings by using effects units. Effects units that are specialized in the generation of the reverberation effect are commonly called reverbs. Read more... -
The fundamental frequency, often referred to simply as the fundamental, is defined as the lowest frequency of a periodic waveform. In music, the fundamental is the musical pitch of a note that is perceived as the lowest partial present. In terms of a superposition of sinusoids (e.g. Fourier series), the fundamental frequency is the lowest frequency sinusoidal in the sum. In some contexts, the fundamental is usually abbreviated as f0 (or FF), indicating the lowest frequency counting from zero. In other contexts, it is more common to abbreviate it as f1, the first harmonic. (The second harmonic is then f2 = 2⋅f1, etc. In this context, the zeroth harmonic would be 0 Hz.) Read more...
A combination tone (also called resultant or subjective tone) is a psychoacoustic phenomenon of an additional tone or tones that are artificially perceived when two real tones are sounded at the same time. Their discovery is credited to the violinist Giuseppe Tartini (although he was not the first, see Sorge) and so are also called Tartini tones.
There are two types of combination tones: sum tones whose frequencies are found by adding the frequencies of the real tones and difference tones whose frequencies are the difference between the frequencies of the real tones. "Combination tones are heard when two pure tones (i.e., tones produced by simple harmonic sound waves having no overtones), differing in frequency by about 50 cycles per second [Hertz] or more, sound together at sufficient intensity."
Combination tones can also be produced electronically by combining two signals in a circuit that has nonlinear distortion, such as an amplifier subject to clipping or a ring modulator. Read more...
Ernst Florens Friedrich Chladni (German: [ˈɛʁnst ˈfloːʁɛns ˈfʁiːdʁɪç ˈkladnɪ]; 30 November 1756 – 3 April 1827) was a German physicist and musician. His most important work, for which he is sometimes labeled the father of acoustics, included research on vibrating plates and the calculation of the speed of sound for different gases. He also undertook pioneering work in the study of meteorites and is regarded by some as the father of meteoritics. Read more...- Acoustic resonance is a phenomenon where acoustic systems amplify sound waves whose frequency matches one of its own natural frequencies of vibration (its resonance frequencies).
The term "acoustic resonance" is sometimes used to narrow mechanical resonance to the frequency range of human hearing, but since acoustics is defined in general terms concerning vibrational waves in matter, acoustic resonance can occur at frequencies outside the range of human hearing.
An acoustically resonant object usually has more than one resonance frequency, especially at harmonics of the strongest resonance. It will easily vibrate at those frequencies, and vibrate less strongly at other frequencies. It will "pick out" its resonance frequency from a complex excitation, such as an impulse or a wideband noise excitation. In effect, it is filtering out all frequencies other than its resonance. Read more... - Musical acoustics or music acoustics is a branch of acoustics concerned with researching and describing the physics of music – how sounds are employed to make music. Examples of areas of study are the function of musical instruments, the human voice (the physics of speech and singing), computer analysis of melody, and in the clinical use of music in music therapy. Read more...
- In audio signal processing and acoustics, echo is a reflection of sound that arrives at the listener with a delay after the direct sound. The delay is directly proportional to the distance of the reflecting surface from the source and the listener. Typical examples are the echo produced by the bottom of a well, by a building, or by the walls of an enclosed room and an empty room. A true echo is a single reflection of the sound source.
The word echo derives from the Greek ἠχώ (ēchō), itself from ἦχος (ēchos), "sound". Echo in the folk story of Greek is a mountain nymph whose ability to speak was cursed, only able to repeat the last words anyone spoke to her. Some animals use echo for location sensing and navigation, such as cetaceans (dolphins and whales) and bats. Read more... - The sound of a violin is the result of interactions between its many parts. Drawing a bow across the strings causes the strings to vibrate. This vibration is transmitted through the bridge and sound post to the body of the violin, which allows the sound to effectively radiate into the surrounding air. The tension and type of strings, placement and tension of the sound post, quality of the bow, and the construction of the body, all contribute to the loudness and tonal quality of the sound. Read more...
- Any signal that can be represented as a variable that varies in time has a corresponding frequency spectrum. This includes familiar entities such as visible light (perceived as color), musical notes (perceived as pitch), radio/TV (specified by their frequency, or sometimes wavelength) and even the regular rotation of the earth. When these signals are viewed in the form of a frequency spectrum, certain aspects of the received signals or the underlying processes producing them are revealed. In some cases the frequency spectrum may include a distinct peak corresponding to a sine wave component. And additionally there may be peaks corresponding to harmonics of a fundamental peak, indicating a periodic signal which is not simply sinusoidal. Or a continuous spectrum may show narrow frequency intervals which are strongly enhanced corresponding to resonances, or frequency intervals containing almost zero power as would be produced by a notch filter.
In physics, the signal might be a wave, such as an electromagnetic wave, an acoustic wave, or the vibration of a mechanism. The power spectral density (PSD) of the signal describes the power present in the signal as a function of frequency, per unit frequency. Power spectral density is commonly expressed in watts per hertz (W/Hz).
When a signal is defined in terms only of a voltage, for instance, there is no unique power associated with the stated amplitude. In this case "power" is simply reckoned in terms of the square of the signal, as this would always be proportional to the actual power delivered by that signal into a given impedance. So one might use units of V2 Hz−1 for the PSD and V2 s Hz−1 for the ESD (energy spectral density) even though no actual "power" or "energy" is specified. Read more... - Jens Peter Blauert (born 20 June 1938 in Hamburg) is a German scientist specializing in psycho-acoustics and an emeritus professor at the Ruhr-Universität Bochum, where he founded the Institute of Communication Acoustics. His major scientific fields of interest are spatial hearing, binaural technology, aural architecture, perceptual quality, speech technology, virtual environments and tele-presence. Read more...
A node is a point along a standing wave where the wave has minimum amplitude. For instance, in a vibrating guitar string, the ends of the string are nodes. By changing the position of the end node through frets, the guitarist changes the effective length of the vibrating string and thereby the note played. The opposite of a node is an anti-node, a point where the amplitude of the standing wave is a maximum. These occur midway between the nodes. Read more...
Ultrasound is sound waves with frequencies higher than the upper audible limit of human hearing. Ultrasound is not different from "normal" (audible) sound in its physical properties, except that humans cannot hear it. This limit varies from person to person and is approximately 20 kilohertz (20,000 hertz) in healthy young adults. Ultrasound devices operate with frequencies from 20 kHz up to several gigahertz.
Ultrasound is used in many different fields. Ultrasonic devices are used to detect objects and measure distances. Ultrasound imaging or sonography is often used in medicine. In the nondestructive testing of products and structures, ultrasound is used to detect invisible flaws. Industrially, ultrasound is used for cleaning, mixing, and accelerating chemical processes. Animals such as bats and porpoises use ultrasound for locating prey and obstacles. Scientists are also studying ultrasound using graphene diaphragms as a method of communication. Read more...- Joseph Sauveur (24 March 1653 – 9 July 1716) was a French mathematician and physicist. He was a professor of mathematics and in 1696 became a member of the French Academy of Sciences. Read more...
A string, tied at A, is kept in tension by W, a suspended weight, and two bridges, B and the movable bridge C, while D is a freely moving wheel, density may be tested by using different strings
A monochord, also known as sonometer (see below), is an ancient musical and scientific laboratory instrument, involving one (mono) string (Chord). The term monochord is sometimes used as the class-name for any musical stringed instrument having only one string and a stick shaped body, also known as musical bows. According to the Hornbostel–Sachs system, string bows are bar zithers (311.1) while monochords are traditionally board zithers (314). The "harmonical canon", or monochord is, at its least, "merely a string having a board under it of exactly the same length, upon which may be delineated the points at which the string must be stopped to give certain notes," allowing comparison.
A string is fixed at both ends and stretched over a sound box. One or more movable bridges are then manipulated to demonstrate mathematical relationships among the frequencies produced. "With its single string, movable bridge and graduated rule, the monochord (kanōn [Greek: law]) straddled the gap between notes and numbers, intervals and ratios, sense-perception and mathematical reason." However, "music, mathematics, and astronomy were [also] inexorably linked in the monochord." As a pedagogical tool for demonstrating mathematical relationships between intervals, the monochord remained in use throughout the middle ages. Read more...- Dr. Dale Vance Holliday (May 29, 1940 – February 4, 2010) was born in Ennis, Texas and attended the University of Texas at Austin. He graduated with a B.S. and M.A. in Physics and did extensive theoretical and experimental research on the Mössbauer effect. He died in San Diego on February 4, 2010 after a period of illness.
Holliday became one of the first 100 employees of Tracor in 1962 and quickly became Director of Analysis and Applied Research in the Electronic Systems Division. In 1965 Holliday left Austin to develop the Tracor facility in San Diego and began his doctorate in applied physics at the University of California, San Diego.
In San Diego Holliday began his study in acoustics for which he is known. His study of transient flow in natural gas pipelines led to the publication of a textbook which is still used as a standard reference. Read more...
Need help?
Do you have a question about Acoustics that you can't find the answer to?
Consider asking it at the Wikipedia reference desk.
Get involved
For editor resources and to collaborate with other editors on improving Wikipedia's Acoustics-related articles, see WikiProject Acoustics.
Selected images
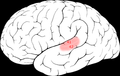
The primary auditory cortex is one of the main areas associated with superior pitch resolution.
Principles of acoustics have been applied since ancient times : A Roman theatre in the city of Amman.
_(9420310527).jpg/80px-Flûte_paléolithique_(musée_national_de_Slovénie%2C_Ljubljana)_(9420310527).jpg)
The Divje Babe flute
Ultrasound image of a fetus in the womb, viewed at 12 weeks of pregnancy (bidimensional-scan)
The fundamental acoustical process
_(9420310527).jpg)
Subcategories
- Select [►] to view subcategories
Subtopics
| Engineering | ||
|---|---|---|
| Psychoacoustics | ||
| Frequency and pitch | ||
| Acousticians | ||
| Related topics | ||
