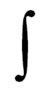Glossary of calculus

Most of the terms listed in Wikipedia glossaries are already defined and explained within Wikipedia itself. However, glossaries like this one are useful for looking up, comparing and reviewing large numbers of terms together. You can help enhance this page by adding new terms or writing definitions for existing ones.

This glossary of calculus is a list of definitions about calculus, its sub-disciplines, and related fields.

Part of a series of articles about
Calculus
Fundamental theorem Limits of functions Continuity Mean value theorem Rolle's theorem
Differential Definitions Derivative (generalizations) Differential infinitesimal of a function total Concepts Differentiation notation Second derivative Third derivative Change of variables Implicit differentiation Related rates Taylor's theorem Rules and identities Sum Product Chain Power Quotient Inverse General Leibniz Faà di Bruno's formula
Integral Lists of integrals Definitions Antiderivative Integral (improper) Riemann integral Lebesgue integration Contour integration Integration by Parts Discs Cylindrical shells Substitution (trigonometric) Partial fractions Order Reduction formulae
Series Geometric (arithmetico-geometric) Harmonic Alternating Power Binomial Taylor Convergence tests Summand limit (term test) Ratio Root Integral Direct comparison Limit comparison Alternating series Cauchy condensation Dirichlet Abel
Vector Gradient Divergence Curl Laplacian Directional derivative Identities Theorems Divergence Gradient Green's Kelvin–Stokes Stokes
Multivariable Formalisms Matrix Tensor Exterior Geometric Definitions Partial derivative Multiple integral Line integral Surface integral Volume integral Jacobian Hessian
Specialized Fractional Malliavin Stochastic Variations
Glossary of calculus Glossary of calculus

Part of a series on
Science
Formal Formal logic Mathematics Mathematical statistics Theoretical computer science Game theory Decision theory Actuarial science Information theory Systems theory Control theory
Physical Physics Classical Modern Applied Theoretical Experimental Computational Mechanics (classical analytical continuum fluid solid) Electromagnetism Thermodynamics Molecular Atomic Nuclear Particle Condensed matter Plasma Quantum mechanics (introduction) Quantum field theory Special relativity General relativity String theory Chemistry Inorganic Organic Analytical Physical Acid-base Supramolecular Solid-state Nuclear Environmental Sustainable ("green") Theoretical Astrochemistry Biochemistry Crystallography Food chemistry Geochemistry Materials science Photochemistry Radiochemistry Stereochemistry Surface science Earth science Climatology Edaphology Environmental science Geodesy Geography (physical) Geology Geomorphology Geophysics Glaciology Hydrology Limnology Meteorology Mineralogy Oceanography Paleoclimatology Paleoecology Palynology Pedology Volcanology Astronomy Astrophysics Cosmology Galactic astronomy Planetary geology Planetary science Stellar astronomy
Life Biology Anatomy Astrobiology Biochemistry Biogeography Biological engineering Biomechanics Biophysics Biotechnology Botany Cell biology Conservation biology Cryobiology Developmental biology Ecology Ethology Evolutionary biology (introduction) Genetics (introduction) Immunology Microbiology Molecular anthropology Molecular biology Nanobiology Neuroscience Paleontology Parasitology Physiology Quantum biology Theoretical biology Toxicology Zoology
Social Anthropology Archaeology Criminology Demography Economics Geography (human) History International relations Jurisprudence Linguistics Pedagogy Political science Psychology Sociology
Applied Engineering Aerospace Agricultural Applied physics Biological Biomedical Chemical Civil Computer science / engineering Electrical Fire protection Genetic Industrial Information Mechanical Military Mining Nuclear Operations research Robotics Software Web Healthcare Medicine Veterinary Dentistry Midwifery Epidemiology Pharmacy Nursing
Interdisciplinary Artificial intelligence Bioethics Bioinformatics Biostatistics Cognitive science Complex systems Computational linguistics Cultural studies Cybernetics Environmental science Environmental social science Environmental studies Ethnic studies Evolutionary psychology Forensics Forestry Library science Mathematical / theoretical biology Mathematical physics Military science Network science Neural engineering Science studies Scientific modelling Semiotics Sociobiology Statistics Systems science Urban planning Web science
Philosophy History Basic research Citizen science Fringe science Protoscience Pseudoscience Freedom Policy Funding Method Sociology Technoscience
Glossaries of science and engineering Aerospace engineering Archaeology Architecture Artificial intelligence Astronomy Biology Botany Calculus Chemistry Civil engineering Clinical research Computer science Ecology Economics Electrical and electronics engineering Engineering Entomology Environmental science Genetics Geography Geology Machine vision Mechanical engineering Medicine Meteoritics Meteorology Physics Probability and statistics Robotics Speciation Structural engineering
Outline Portal Category

A

Abel's test

A method of testing for the convergence of an infinite series.

Absolute convergence

An infinite series of numbers is said to converge absolutely (or to be absolutely convergent) if the sum of the absolute values of the summands is finite. More precisely, a real or complex series $\textstyle \sum _{n=0}^{\infty }a_{n}$ is said to converge absolutely if $\textstyle \sum _{n=0}^{\infty }\left|a_{n}\right|=L$ for some real number $\textstyle L$. Similarly, an improper integral of a function, $\textstyle \int _{0}^{\infty }f(x)\,dx$, is said to converge absolutely if the integral of the absolute value of the integrand is finite—that is, if $\textstyle \int _{0}^{\infty }\left|f(x)\right|dx=L.$

Absolute maximum

Absolute minimum

Absolute value

The absolute value or modulus |x| of a real number x is the non-negative value of x without regard to its sign. Namely, |x| = x for a positive x, |x| = −x for a negative x (in which case −x is positive), and |0| = 0. For example, the absolute value of 3 is 3, and the absolute value of −3 is also 3. The absolute value of a number may be thought of as its distance from zero.

Alternating series

An infinite series whose terms alternate between positive and negative.

Alternating series test

Is the method used to prove that an alternating series with terms that decrease in absolute value is a convergent series. The test was used by Gottfried Leibniz and is sometimes known as Leibniz's test, Leibniz's rule, or the Leibniz criterion.

Annulus

A ring-shaped object, a region bounded by two concentric circles.

Antiderivative

An antiderivative, primitive function, primitive integral or indefinite integral of a function f is a differentiable function F whose derivative is equal to the original function f. This can be stated symbolically as $F'=f$. The process of solving for antiderivatives is called antidifferentiation (or indefinite integration) and its opposite operation is called differentiation, which is the process of finding a derivative.

Arcsin

Area under a curve

Asymptote

In analytic geometry, an asymptote of a curve is a line such that the distance between the curve and the line approaches zero as one or both of the x or y coordinates tends to infinity. Some sources include the requirement that the curve may not cross the line infinitely often, but this is unusual for modern authors. In projective geometry and related contexts, an asymptote of a curve is a line which is tangent to the curve at a point at infinity.

Automatic differentiation

In mathematics and computer algebra, automatic differentiation (AD), also called algorithmic differentiation or computational differentiation, is a set of techniques to numerically evaluate the derivative of a function specified by a computer program. AD exploits the fact that every computer program, no matter how complicated, executes a sequence of elementary arithmetic operations (addition, subtraction, multiplication, division, etc.) and elementary functions (exp, log, sin, cos, etc.). By applying the chain rule repeatedly to these operations, derivatives of arbitrary order can be computed automatically, accurately to working precision, and using at most a small constant factor more arithmetic operations than the original program.

Average rate of change

B

Binomial coefficient

Any of the positive integers that occurs as a coefficient in the binomial theorem is a binomial coefficient. Commonly, a binomial coefficient is indexed by a pair of integers n ≥ k ≥ 0 and is written ${\tbinom {n}{k}}.$ It is the coefficient of the x^k term in the polynomial expansion of the binomial power (1 + x)ⁿ, and it is given by the formula

${\binom {n}{k}}={\frac {n!}{k!(n-k)!}}.$

Binomial theorem (or binomial expansion)

Describes the algebraic expansion of powers of a binomial.

Bounded function

A function f defined on some set X with real or complex values is called bounded, if the set of its values is bounded. In other words, there exists a real number M such that

$|f(x)|\leq M$

for all x in X. A function that is not bounded is said to be unbounded. Sometimes, if f(x) ≤ A for all x in X, then the function is said to be bounded above by A. On the other hand, if f(x) ≥ B for all x in X, then the function is said to be bounded below by B.

Bounded sequence

.

C

Calculus

(From Latin calculus, literally 'small pebble', used for counting and calculations, as on an abacus) is the mathematical study of continuous change, in the same way that geometry is the study of shape and algebra is the study of generalizations of arithmetic operations.

Cavalieri's principle

In geometry, Cavalieri's principle, a modern implementation of the method of indivisibles, named after Bonaventura Cavalieri, is as follows:

• 2-dimensional case: Suppose two regions in a plane are included between two parallel lines in that plane. If every line parallel to these two lines intersects both regions in line segments of equal length, then the two regions have equal areas.
• 3-dimensional case: Suppose two regions in three-space (solids) are included between two parallel planes. If every plane parallel to these two planes intersects both regions in cross-sections of equal area, then the two regions have equal volumes.

Chain rule

The chain rule is a formula for computing the derivative of the composition of two or more functions. That is, if f and g are functions, then the chain rule expresses the derivative of their composition f ∘ g (the function which maps x to f(g(x)) ) in terms of the derivatives of f and g and the product of functions as follows:

$(f\circ g)'=(f'\circ g)\cdot g'.$

This may equivalently be expressed in terms of the variable. Let F = f ∘ g, or equivalently, F(x) = f(g(x)) for all x. Then one can also write

$F'(x)=f'(g(x))g'(x).$

The chain rule may be written in Leibniz's notation in the following way. If a variable z depends on the variable y, which itself depends on the variable x, so that y and z are therefore dependent variables, then z, via the intermediate variable of y, depends on x as well. The chain rule then states,

${\frac {dz}{dx}}={\frac {dz}{dy}}\cdot {\frac {dy}{dx}}.$

The two versions of the chain rule are related; if $z=f(y)$ and $y=g(x)$, then

${\frac {dz}{dx}}={\frac {dz}{dy}}\cdot {\frac {dy}{dx}}=f'(y)g'(x)=f'(g(x))g'(x).$

In integration, the counterpart to the chain rule is the substitution rule.

Change of variables

Is a basic technique used to simplify problems in which the original variables are replaced with functions of other variables. The intent is that when expressed in new variables, the problem may become simpler, or equivalent to a better understood problem.

Cofunction

A function f is cofunction of a function g if f(A) = g(B) whenever A and B are complementary angles. This definition typically applies to trigonometric functions. The prefix "co-" can be found already in Edmund Gunter's Canon triangulorum (1620). .

Concave function

Is the negative of a convex function. A concave function is also synonymously called concave downwards, concave down, convex upwards, convex cap or upper convex.

Constant of integration

The indefinite integral of a given function (i.e., the set of all antiderivatives of the function) on a connected domain is only defined up to an additive constant, the constant of integration. This constant expresses an ambiguity inherent in the construction of antiderivatives. If a function $f(x)$ is defined on an interval and $F(x)$ is an antiderivative of $f(x)$, then the set of all antiderivatives of $f(x)$ is given by the functions $F(x)+C$, where C is an arbitrary constant (meaning that any value for C makes $F(x)+C$ a valid antiderivative). The constant of integration is sometimes omitted in lists of integrals for simplicity.

Continuous function

Is a function for which sufficiently small changes in the input result in arbitrarily small changes in the output. Otherwise, a function is said to be a discontinuous function. A continuous function with a continuous inverse function is called a homeomorphism.

Continuously differentiable

A function f is said to be continuously differentiable if the derivative f′(x) exists and is itself a continuous function.

Contour integration

In the mathematical field of complex analysis, contour integration is a method of evaluating certain integrals along paths in the complex plane.

Convergence tests

Are methods of testing for the convergence, conditional convergence, absolute convergence, interval of convergence or divergence of an infinite series $\sum _{n=1}^{\infty }a_{n}$.

Convergent series

In mathematics, a series is the sum of the terms of an infinite sequence of numbers. Given an infinite sequence $\left(a_{1},\ a_{2},\ a_{3},\dots \right)$, the nth partial sum $S_{n}$ is the sum of the first n terms of the sequence, that is,

$S_{n}=\sum _{k=1}^{n}a_{k}.$

A series is convergent if the sequence of its partial sums $\left\{S_{1},\ S_{2},\ S_{3},\dots \right\}$ tends to a limit; that means that the partial sums become closer and closer to a given number when the number of their terms increases. More precisely, a series converges, if there exists a number $\ell$ such that for any arbitrarily small positive number $\varepsilon$, there is a (sufficiently large) integer $N$ such that for all $n\geq \ N$,

$\left|S_{n}-\ell \right\vert \leq \ \varepsilon .$

If the series is convergent, the number $\ell$ (necessarily unique) is called the sum of the series. Any series that is not convergent is said to be divergent.

Convex function

In mathematics, a real-valued function defined on an n-dimensional interval is called convex (or convex downward or concave upward) if the line segment between any two points on the graph of the function lies above or on the graph, in a Euclidean space (or more generally a vector space) of at least two dimensions. Equivalently, a function is convex if its epigraph (the set of points on or above the graph of the function) is a convex set. For a twice differentiable function of a single variable, if the second derivative is always greater than or equal to zero for its entire domain then the function is convex. Well-known examples of convex functions include the quadratic function $x^{2}$ and the exponential function $e^{x}$.

Cramer's rule

In linear algebra, Cramer's rule is an explicit formula for the solution of a system of linear equations with as many equations as unknowns, valid whenever the system has a unique solution. It expresses the solution in terms of the determinants of the (square) coefficient matrix and of matrices obtained from it by replacing one column by the column vector of right-hand-sides of the equations. It is named after Gabriel Cramer (1704–1752), who published the rule for an arbitrary number of unknowns in 1750, although Colin Maclaurin also published special cases of the rule in 1748 (and possibly knew of it as early as 1729)..

Critical point

A critical point or stationary point of a differentiable function of a real or complex variable is any value in its domain where its derivative is 0.

Curve

A curve (also called a curved line in older texts) is, generally speaking, an object similar to a line but that need not be straight.

Curve sketching

In geometry, curve sketching (or curve tracing) includes techniques that can be used to produce a rough idea of overall shape of a plane curve given its equation without computing the large numbers of points required for a detailed plot. It is an application of the theory of curves to find their main features. Here input is an equation. In digital geometry it is a method of drawing a curve pixel by pixel. Here input is an array ( digital image).

D

Damped sine wave

Is a sinusoidal function whose amplitude approaches zero as time increases.

Degree of a polynomial

Is the highest degree of its monomials (individual terms) with non-zero coefficients. The degree of a term is the sum of the exponents of the variables that appear in it, and thus is a non-negative integer.

Derivative

The derivative of a function of a real variable measures the sensitivity to change of the function value (output value) with respect to a change in its argument (input value). Derivatives are a fundamental tool of calculus. For example, the derivative of the position of a moving object with respect to time is the object's velocity: this measures how quickly the position of the object changes when time advances.

Derivative test

A derivative test uses the derivatives of a function to locate the critical points of a function and determine whether each point is a local maximum, a local minimum, or a saddle point. Derivative tests can also give information about the concavity of a function.

Differentiable function

A differentiable function of one real variable is a function whose derivative exists at each point in its domain. As a result, the graph of a differentiable function must have a (non-vertical) tangent line at each point in its domain, be relatively smooth, and cannot contain any breaks, bends, or cusps.

Differential (infinitesimal)

The term differential is used in calculus to refer to an infinitesimal (infinitely small) change in some varying quantity. For example, if x is a variable, then a change in the value of x is often denoted Δx (pronounced delta x). The differential dx represents an infinitely small change in the variable x. The idea of an infinitely small or infinitely slow change is extremely useful intuitively, and there are a number of ways to make the notion mathematically precise. Using calculus, it is possible to relate the infinitely small changes of various variables to each other mathematically using derivatives. If y is a function of x, then the differential dy of y is related to dx by the formula

$dy={\frac {dy}{dx}}\,dx,$

where dy/dx denotes the derivative of y with respect to x. This formula summarizes the intuitive idea that the derivative of y with respect to x is the limit of the ratio of differences Δy/Δx as Δx becomes infinitesimal.

Differential calculus

Is a subfield of calculus concerned with the study of the rates at which quantities change. It is one of the two traditional divisions of calculus, the other being integral calculus, the study of the area beneath a curve.

Differential equation

Is a mathematical equation that relates some function with its derivatives. In applications, the functions usually represent physical quantities, the derivatives represent their rates of change, and the equation defines a relationship between the two.

Differential operator

.

Differential of a function

In calculus, the differential represents the principal part of the change in a function y = f(x) with respect to changes in the independent variable. The differential dy is defined by

$dy=f'(x)\,dx,$

where $f'(x)$ is the derivative of f with respect to x, and dx is an additional real variable (so that dy is a function of x and dx). The notation is such that the equation

$dy={\frac {dy}{dx}}\,dx$

holds, where the derivative is represented in the Leibniz notation dy/dx, and this is consistent with regarding the derivative as the quotient of the differentials. One also writes

$df(x)=f'(x)\,dx.$

The precise meaning of the variables dy and dx depends on the context of the application and the required level of mathematical rigor. The domain of these variables may take on a particular geometrical significance if the differential is regarded as a particular differential form, or analytical significance if the differential is regarded as a linear approximation to the increment of a function. Traditionally, the variables dx and dy are considered to be very small (infinitesimal), and this interpretation is made rigorous in non-standard analysis.

Differentiation rules

.

Direct comparison test

A convergence test in which an infinite series or an improper integral is compared to one with known convergence properties.

Dirichlet's test

Is a method of testing for the convergence of a series. It is named after its author Peter Gustav Lejeune Dirichlet, and was published posthumously in the Journal de Mathématiques Pures et Appliquées in 1862. The test states that if $\{a_{n}\}$ is a sequence of real numbers and $\{b_{n}\}$ a sequence of complex numbers satisfying

• $a_{n+1}\leq a_{n}$

• $\lim _{n\rightarrow \infty }a_{n}=0$

• $\left|\sum _{n=1}^{N}b_{n}\right|\leq M$ for every positive integer N

where M is some constant, then the series

$\sum _{n=1}^{\infty }a_{n}b_{n}$

converges.

Disc integration

.

Divergent series

.

Discontinuity

.

Dot product

.

Double integral

E

• e (mathematical constant)
• Elliptic integral
• Essential discontinuity
• Euler method
• Exponential function
• Extreme value theorem
• Extremum

F

Faà di Bruno's formula

First-degree polynomial

First derivative test

Fractional calculus

Frustum

Function

Function composition

Fundamental theorem of calculus

The fundamental theorem of calculus is a theorem that links the concept of differentiating a function with the concept of integrating a function. The first part of the theorem, sometimes called the first fundamental theorem of calculus, states that one of the antiderivatives (also called indefinite integral), say F, of some function f may be obtained as the integral of f with a variable bound of integration. This implies the existence of antiderivatives for continuous functions. Conversely, the second part of the theorem, sometimes called the second fundamental theorem of calculus, states that the integral of a function f over some interval can be computed by using any one, say F, of its infinitely many antiderivatives. This part of the theorem has key practical applications, because explicitly finding the antiderivative of a function by symbolic integration avoids numerical integration to compute integrals. This provides generally a better numerical accuracy.

G

• General Leibniz rule
• Global maximum
• Global minimum
• Golden spiral
• Gradient

H

Harmonic progression

In mathematics, a harmonic progression (or harmonic sequence) is a progression formed by taking the reciprocals of an arithmetic progression. It is a sequence of the form

${\frac {1}{a}},\ {\frac {1}{a+d}}\ ,{\frac {1}{a+2d}}\ ,{\frac {1}{a+3d}}\ ,\cdots ,{\frac {1}{a+kd}},$

where −a/d is not a natural number and k is a natural number. Equivalently, a sequence is a harmonic progression when each term is the harmonic mean of the neighboring terms. It is not possible for a harmonic progression (other than the trivial case where a = 1 and k = 0) to sum to an integer. The reason is that, necessarily, at least one denominator of the progression will be divisible by a prime number that does not divide any other denominator.

Higher derivative

.

Homogeneous linear differential equation

.

Hyperbolic function

.

I

• Identity function
• Imaginary number
• Implicit function
• Improper fraction
• Improper integral
• Inflection point - a point at which a plane curve's concavity changes.
• Instantaneous rate of change
• Instantaneous velocity
• Integral
• Integral symbol
• Integrand - the function to be integrated in an integral.
• Integration by parts
• Integration by substitution
• Intermediate value theorem
• Inverse trigonometric functions

J

• Jump discontinuity

K

L

• Law of cosines
• Law of sines
• Lebesgue integration
• L'Hôpital's rule
• Limit comparison test
• Limit of a function
• Limits of integration
• Linear combination
• Linear equation
• Linear system
• List of integrals
• Logarithm
• Logarithmic differentiation
• Lower bound

M

• Mean value theorem
• Monotonic function
• Multiple integral
• Multiplicative calculus
• Multivariable calculus

N

• Natural logarithm - The natural logarithm of a number is its logarithm to the base of the mathematical constant e, where e is an irrational and transcendental number approximately equal to 7000271828182845899♠2.718281828459. The natural logarithm of x is generally written as ln x, log_e x, or sometimes, if the base e is implicit, simply log x. Parentheses are sometimes added for clarity, giving ln(x), log_e(x) or log(x). This is done in particular when the argument to the logarithm is not a single symbol, to prevent ambiguity.
• Non-Newtonian calculus
• Nonstandard calculus
• Notation for differentiation
• Numerical integration

O

• One-sided limit
• Ordinary differential equation

P

• Pappus's centroid theorem
• Parabola
• Paraboloid
• Partial derivative
• Partial differential equation
• Partial fraction decomposition
• Particular solution
• Piecewise-defined function - a function defined by multiple sub-functions that apply to certain intervals of the function's domain.
• Position vector
• Power rule
• Product integral
• Product rule
• Proper fraction
• Proper rational function
• Pythagorean theorem
• Pythagorean trigonometric identity

Q

• Quadratic function
• Quadratic polynomial
• Quotient rule - a formula for finding the derivative of a function that is the ratio of two functions.

R

• Radian
• Ratio test
• Reciprocal function
• Reciprocal rule
• Riemann integral
• Related rates
• Removable discontinuity
• Rolle's theorem
• Root test

S

• Scalar
• Secant line
• Second-degree polynomial
• Second derivative
• Second derivative test
• Second-order differential equation
• Series
• Shell integration
• Simpson's rule
• Sine
• Sine wave
• Slope field
• Squeeze theorem
• Sum rule in differentiation
• Sum rule in integration
• Summation
• Supplementary angle
• Surface area
• System of linear equations

T

• Table of integrals
• Taylor series
• Taylor's theorem
• Tangent
• Third-degree polynomial
• Third derivative
• Toroid
• Total differential
• Trigonometric functions
• Trigonometric identities
• Trigonometric integral
• Trigonometric substitution
• Trigonometry
• Triple integral

U

• Upper bound

V

• Variable
• Vector
• Vector calculus

W

• Washer
• Washer method

X

Y

Z

• Zero vector

See also

• Calculus
• Outline of calculus
• Glossary of areas of mathematics
• Glossary of astronomy
• Glossary of biology
• Glossary of botany
• Glossary of chemistry
• Glossary of ecology
• Glossary of engineering
• Glossary of physics
• Glossary of probability and statistics

References

Notes