Charge qubit

From Wikipedia, the free encyclopedia
Jump to navigation Jump to search
Circuit diagram of a Cooper pair box circuit. The island (dotted line) is formed by the superconducting electrode between the gate capacitor and the junction capacitance.

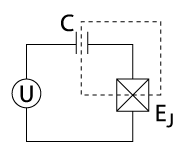
In quantum computing, a charge qubit is a qubit whose basis states are charge states (e.g. states which represent the presence or absence of excess Cooper pairs in the island).[1][2][3] In superconducting quantum computing, a charge qubit is formed by a tiny superconducting island (also known as a Cooper-pair box) coupled by a Josephson junction to a superconducting reservoir (see figure). The state of the qubit is determined by the number of Cooper pairs which have tunneled across the junction. In contrast with the charge state of an atomic or molecular ion, the charge states of such an "island" involve a macroscopic number of conduction electrons of the island. The quantum superposition of charge states can be achieved by tuning the gate voltage U that controls the chemical potential of the island. The charge qubit is typically read-out by electrostatically coupling the island to an extremely sensitive electrometer such as the radio-frequency single-electron transistor.

Typical T2 coherence times for a charge qubit are on the order of 1–2 μs.[4] Recent work has shown T2 times approaching 100 μs using a type of charge qubit known as a transmon inside a three-dimensional superconducting cavity.[5][6] Understanding the limits of T2 is an active area of research in the field of superconducting quantum computing.

Benefits[edit]

To-date, the realizations of qubits that have had the most success are ion traps and NMR, with Shor's algorithm even being implemented using NMR.[7] However, it is hard to see these two methods being scaled to the hundreds, thousands, or millions of qubits necessary to create a quantum computer. Solid-state representations of qubits are much more easily scalable, but they themselves have their own problem: decoherence. Superconductors, however, have the advantage of being more easily scaled, and they are more coherent than normal solid-state systems.[8]

Experimental Progress[edit]

Superconducting charge qubits have been progressing quickly. They were first suggested in 1997 by Shnirman,[9] and by 2001 coherent oscillations were observed.

References[edit]

  1. ^ V. Bouchiat, D. Vion, P. Joyez, D. Esteve and M. H. Devoret, "Quantum coherence with a single Cooper pair", Physica Scripta T76, 165-170 (1998), doi:10.1238/Physica.Topical.076a00165
  2. ^ Y. Nakamura, Yu. A. Pashkin and J. S. Tsai, "Coherent control of macroscopic quantum states in a single-Cooper-pair box", Nature 398, 786-788 (1999), doi:10.1038/19718, arXiv:9904003
  3. ^ K. W. Lehnert, B. A. Turek, K. Bladh, L. F. Spietz, D. Gunnarsson, P. Delsing and R. J. Schoelkopf, "Measurement of the excited-state lifetime of a microelectronic circuit", Phys. Rev. Lett. 90, 027002 (2003), doi:10.1103/PhysRevLett.90.027002
  4. ^ A.A. Houck et al., "Life after charge noise: recent results with transmon qubits," Quant. Inf. Proc. 8, 105 (2009), doi:10.1007/s11128-009-0100-6, arXiv:0812.1865
  5. ^ H. Paik et al., "Observation of high coherence in Josephson junction qubits measured in a three-dimensional circuit QED architecture," Phys. Rev. Lett. 107, 240501 (2011), doi:10.1103/PhysRevLett.107.240501, arXiv:1105.4652
  6. ^ C. Rigetti et al., "Superconducting qubit in waveguide cavity with coherence time approaching 0.1 ms," arXiv:1202.5533 (2012)
  7. ^ Superconducting Charge Qubits, by Denzil Anthony Rodrigues, page 3
  8. ^ Superconducting Charge Qubits by Denzil Anthony Rodrigues, page 3
  9. ^ A. Shnirman, G. Schön, and Z. Herman, Phys. Rev. Lett. 79, 2371 (1997)
Retrieved from "https://en.wikipedia.org/w/index.php?title=Charge_qubit&oldid=876152611"